SYMMETRY BREAKING IN TORUS KNOTS
SMALL TORUS KNOTS
Let us consider
one of the simplest type of prime knots: torus T2,n knots, whose
n-fold symmetrical conformations are easily defined parametrically. For
n=3, i.e. the 31 (trefoil) knot, its final, tight conformation
preserves the 3-fold symmetry of the initial conformation. For n=5, i.e.
the 51 knot, the 5-fold symmetry of the initial conformation
becomes broken during the tightening process. See figure below.


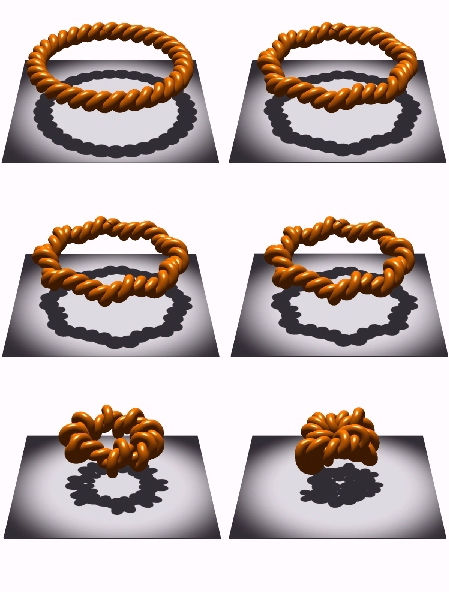
LARGE TORUS KNOTS
For much larger n, the evolution of
the symmetrical, parametrically defined torus knots towards their most
tight conformations is a complex process. Figures below show its consecutive
stages in the cases of n=33 and n=99.
n=33

n=99
Further simulations performed
with larger T2,n torus knots prove that the shapeless, blob-like
conformation shown at the last frame above is not the best one. Starting
from a different initial conformation of the knot we find a different final
conformation, which proves to be slightly better from the point of view
of the length of the rope used to tie it. See below.
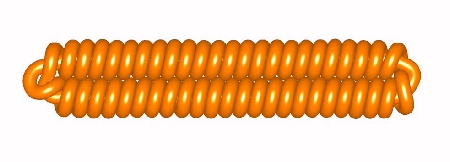
Initial linear conformation
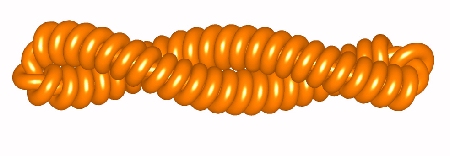
Twisted final conformation
OPEN QUESTION: Are there any better
conformations?
The problem of ideal T2,n
torus knots is closely related to the problem of the ideal twisted pair.
Back to the index page







